1. 
2. Help
3. Log In

Work from transport processes

Example 1:

Mechanism of nigericin

1) Draw out a reaction scheme

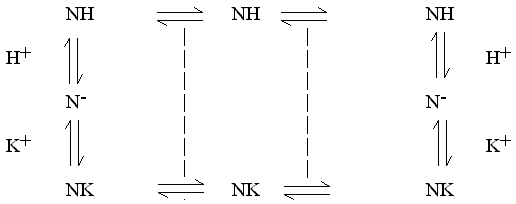
2) For each reaction, write an equation. For a transport process, make sure that you distinguish between reactants in different phases (we use subscripts L, M and R here to denote reactants in the left aqueous, membrane, and right aqueous phases). It is formally correct to write the equations with a direction (reactants --> products) to represent the flux of the transport cycle. Choose a direction for the reaction cycle. For example, if K⁺ is at higher activity on the left, the reaction will lead to K⁺ flow from left to right.

3) At this point, if we have got our directions right, we can sum the reactions, and cancel terms. We will find that all the terms for the carrier cancel, leaving only the components in flux.

4) Now, we can write the free energy equation for the remaining reaction, and then sum the G values to find the overall free energy for the transport process, using:

Then, remembering that by convention, reactants have a negative sign (because their concentration decreases when the reaction proceeds in the direction shown), and substituting from

we get for the above process:

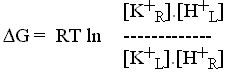
5) We can find the condition for equilibrium by setting G' = 0 :

Example 2.

Mechanism of the ATP^- / ADP carrier (adenine nucleotide translocase).

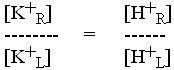
The carrier mechanism catalyzes the obligate exchange of 1 ATP^- for 1 ADP. Although we don't know the mechanism, we can assume (as we found above) that the carrier components cancel out, leaving the components in flux. This will give:

where µ values are chemical potentials, and µ values are electrochemical potentials. The later are chosen for ATP because ATP^- is effectively transported with 1 excess negative charge over ADP.

Substituting from: µ_i' = µ_i^o + RT ln a_i, and from µ_i = µ_i' + zFy

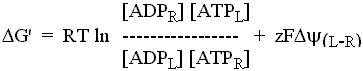
When G' = 0,

The equilibrium case

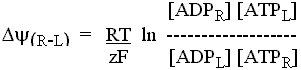
Note that when we assume that a chemical or transport process has reached equilibrium (G' = 0), we can simplify the treatment.

For any reaction at equilibrium, we can simply substitute into the reaction equation, chemical or electrochemical (for charged species moving between phases) potentials for each species, and replace the reaction sign by an equals sign.

For example 1:

and for example 2:

Expansion of the equations then gives the equilibrium equations already derived.